When planning for roofing projects, whether it’s for a new construction or a renovation, knowing the correct area of your roof is essential. Calculating the roof area helps in estimating the amount of material required (such as shingles, tiles, or roofing sheets) and ensures that you purchase enough to cover the entire surface. It also helps when estimating costs for the project. In this guide, we’ll show you how to calculate the area of a roof, step by step, including examples and tips for different roof types.
Step 1: Understand the Roof Type
Before starting the calculations, it’s crucial to determine the type of roof you have, as this will influence the method used. There are several common roof types, and each requires a slightly different approach to measuring.
Common Roof Types:
- Flat Roof: A roof that is nearly horizontal, with little to no pitch.
- Gable Roof: A roof with two sloping sides that meet at a ridge.
- Hip Roof: A roof with slopes on all four sides.
- Mansard Roof: A roof with two slopes on each side, with the lower slope being steeper than the upper one.
- Shed Roof: A simple, single-slope roof.
- Butterfly Roof: A modern roof with two slopes that meet in the middle.
The method of calculation will vary slightly depending on whether the roof is sloped or flat.
Step 2: Measure the Dimensions of the Roof
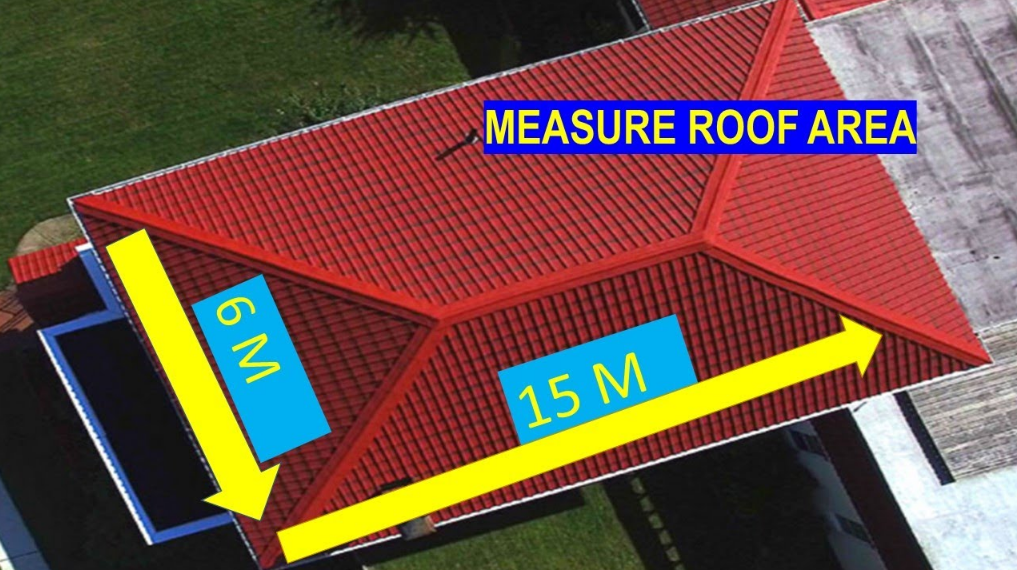
To calculate the area of the roof, you will need to measure the dimensions of the roof structure. Start by measuring the length and width of the roof’s base, followed by measuring the height of the roof’s peak (for sloped roofs).
For a Flat Roof:
- Measure the length and width of the roof at its base. These are straightforward measurements.Example:
- Length of the roof = 10 metres
- Width of the roof = 8 metres
- Area = Length × Width
- Area = 10 m × 8 m = 80 square metres (m²)
For a Sloped Roof (Gable, Hip, Mansard, etc.):
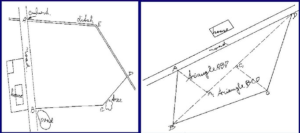
For sloped roofs, you need to calculate the pitch or slope to find the actual surface area. The pitch of the roof refers to how steep the roof is.
- Pitch is typically expressed as the ratio of vertical rise to horizontal run (rise/run).
- You can find the roof’s slope length (the hypotenuse of the triangle formed by the rise and run) using the Pythagorean theorem:
- Slope length = √(rise² + run²)
Step 3: Calculate the Roof Slope (for Sloped Roofs)
For sloped roofs, you need to measure the rise (height) and run (half the length of the building if the roof is symmetrical).
Example Calculation for a Gable Roof:
- Measure the rise and run:
- Rise = 3 metres (height of the peak from the base)
- Run = 4 metres (half of the roof length)
- Find the slope length using the Pythagorean theorem:
- Slope length = √(rise² + run²)
- Slope length = √(3² + 4²) = √(9 + 16) = √25 = 5 metres
- Calculate the area of one side of the roof:
- Area = Length × Slope length
- Area = 10 m × 5 m = 50 m² (for one side)
- Multiply by 2 for both sides (for a symmetrical gable roof):
- Total area = 50 m² × 2 = 100 m²
So, the total area of a gable roof with these dimensions would be 100 m².
Step 4: Adjust for Complex Roofs
Some roofs may have additional features, such as dormers, valleys, or multiple sections. In these cases, it’s best to break the roof down into smaller sections and calculate the area of each section separately.
For a Hip Roof:
A hip roof has four slopes. You will need to calculate the area of each of the four roof sections.
- Measure the dimensions of each slope: Measure the length and width of each sloped section, and calculate the area of each one separately.
- Add the areas together: Add up the areas of the four sections to find the total roof area.
Step 5: Account for Overhangs and Eaves
If the roof has overhangs (the part of the roof that extends beyond the walls of the building), you need to measure the length and width of the overhangs as well, and include them in your total area.
Example for Overhangs:
If the roof has a 1-metre overhang on all sides, add 1 metre to both the length and width of the roof dimensions for each side.
- Length = 10 metres (plus 1 metre for the overhang) = 11 metres
- Width = 8 metres (plus 1 metre for the overhang) = 9 metres
Then recalculate the area:
- Area = Length × Width
- Area = 11 m × 9 m = 99 m²
This gives you the total area of the roof with the overhang included.
Step 6: Consider Roof Pitch Adjustment (for Materials)
If you are planning to use specific roofing materials, it’s important to adjust the calculations based on the pitch. The steeper the pitch, the more material you will need. Some roofing materials, such as shingles or tiles, require adjustments based on the roof’s pitch.
For example, you may need to apply a pitch factor. A common adjustment is to add 10-20% extra material for steeper roofs, depending on the slope.
Example 1: Simple Gable Roof with Overhangs
- Length: 10 metres
- Width: 8 metres
- Rise: 3 metres
- Run: 4 metres
- Overhang: 1 metre on each side
Step-by-Step Calculation:
- Calculate the slope length using the Pythagorean theorem:
- Slope length = √(3² + 4²) = √25 = 5 metres
- Calculate the area for one side of the roof:
- Area = Length × Slope length = 10 m × 5 m = 50 m²
- Multiply by 2 for both sides:
- Total area = 50 m² × 2 = 100 m²
- Add the overhang:
- New Length = 10 m + 1 m = 11 m
- New Width = 8 m + 1 m = 9 m
- Total area with overhang = 11 m × 9 m = 99 m²
So, the total roof area is 99 m².
Calculating the area of a roof is an essential step in any roofing project, whether you’re replacing the roof, planning for insulation, or determining material needs. By understanding the type of roof you have, measuring the dimensions accurately, and considering elements like slope, overhangs, and pitch, you can get a precise measurement of your roof area. For complex roofs, break them down into smaller sections and add up the areas accordingly. Using these steps ensures that you account for all variables and avoid costly mistakes.